Control Canonical Form
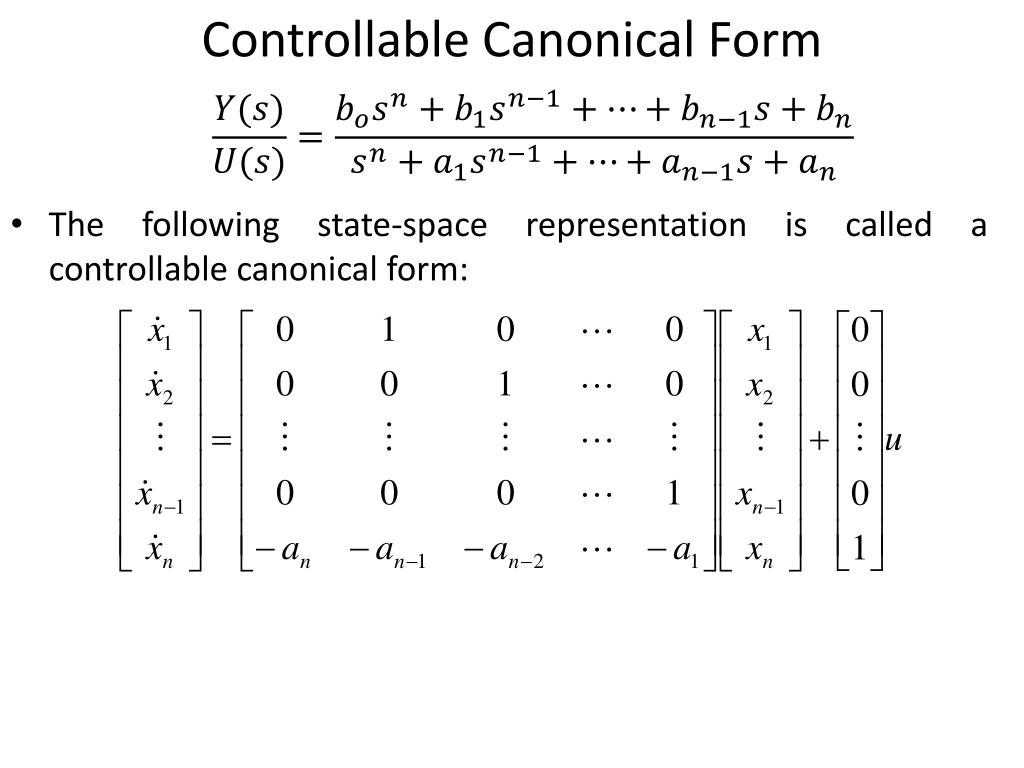
Control Canonical Form - For systems written in control canonical form: Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Instead, the result is what is known as the controller canonical form. Controllable canonical form is a minimal realization in which all model states are controllable. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. This is still a companion form because the coefficients of the. Y = cx is said to be incontroller canonical form(ccf) is the. This form is called the controllable canonical form (for reasons that we will see later). Note how the coefficients of the transfer function show up in.
Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Y = cx is said to be incontroller canonical form(ccf) is the. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. For systems written in control canonical form: This form is called the controllable canonical form (for reasons that we will see later). This is still a companion form because the coefficients of the. Instead, the result is what is known as the controller canonical form. Note how the coefficients of the transfer function show up in. Controllable canonical form is a minimal realization in which all model states are controllable.
Instead, the result is what is known as the controller canonical form. This is still a companion form because the coefficients of the. Controllable canonical form is a minimal realization in which all model states are controllable. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. For systems written in control canonical form: Note how the coefficients of the transfer function show up in. This form is called the controllable canonical form (for reasons that we will see later). Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Y = cx is said to be incontroller canonical form(ccf) is the.
Feedback Control Systems (FCS) ppt download
Instead, the result is what is known as the controller canonical form. Controllable canonical form is a minimal realization in which all model states are controllable. Note how the coefficients of the transfer function show up in. Y = cx is said to be incontroller canonical form(ccf) is the. This is still a companion form because the coefficients of the.
Easy Explanation of Controllable Canonical Form Control Engineering
Instead, the result is what is known as the controller canonical form. Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Controllable canonical form is a minimal realization in which all model states are controllable. Note how the coefficients of the transfer function show up in. For systems written in control.
PPT Feedback Control Systems (FCS) PowerPoint Presentation, free
This is still a companion form because the coefficients of the. This form is called the controllable canonical form (for reasons that we will see later). Instead, the result is what is known as the controller canonical form. Note how the coefficients of the transfer function show up in. Controllable canonical form is a minimal realization in which all model.
(PDF) A Control Canonical Form for Augmented MultiInput Linear Time
Y = cx is said to be incontroller canonical form(ccf) is the. This form is called the controllable canonical form (for reasons that we will see later). Controllable canonical form is a minimal realization in which all model states are controllable. For systems written in control canonical form: Note how the coefficients of the transfer function show up in.
State Space Introduction Controllable Canonical Form YouTube
Y = cx is said to be incontroller canonical form(ccf) is the. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Note how the coefficients of the transfer function show up in. Two companion forms are.
Controllable Canonical Phase Variable Form Method 1 Converting
For systems written in control canonical form: Instead, the result is what is known as the controller canonical form. Note how the coefficients of the transfer function show up in. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+.
Control Theory Derivation of Controllable Canonical Form
Y = cx is said to be incontroller canonical form(ccf) is the. Instead, the result is what is known as the controller canonical form. Note how the coefficients of the transfer function show up in. Controllable canonical form is a minimal realization in which all model states are controllable. This is still a companion form because the coefficients of the.
Solved Consider the system defined by * = AX + Bu = Cx where
Controllable canonical form is a minimal realization in which all model states are controllable. For systems written in control canonical form: Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Two companion forms are convenient to.
LCS 53a Controllable Canonical Form (CCF) statespace models YouTube
Instead, the result is what is known as the controller canonical form. Note how the coefficients of the transfer function show up in. Controllable canonical form is a minimal realization in which all model states are controllable. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2.
PPT Feedback Control Systems (FCS) PowerPoint Presentation, free
Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. This form is called the controllable canonical form (for reasons that we will see later). Controllable canonical form is a minimal realization in which all model states.
For Systems Written In Control Canonical Form:
Two companion forms are convenient to use in control theory, namely the observable canonical form and the controllable. Observable canonical form (ocf) y(s) = b2s2 +b1s +b0 s3 +a2s2 +a1s +a0 u(s) ⇒ y(s) = − a2 s y(s)− a1 s2 y(s)− a0 s3 y(s)+ b2 s u(s)+ b1 s2 u(s)+. Instead, the result is what is known as the controller canonical form. Controllable canonical form is a minimal realization in which all model states are controllable.
This Is Still A Companion Form Because The Coefficients Of The.
Note how the coefficients of the transfer function show up in. This form is called the controllable canonical form (for reasons that we will see later). Y = cx is said to be incontroller canonical form(ccf) is the.
.jpg)